Given that, radius $ = \text{OA} = \text{OB} = 1 \; \text{cm},$ and $ \boxed{\text{OC} = \text{OD}} $
So, the $\triangle \text{OCD},$ is isosceles triangle.
An isosceles triangle is a triangle that :
- Have two sides equal
- The base angles are also equal
- The perpendicular from the apex angle bisects the base
We can draw the diagram,
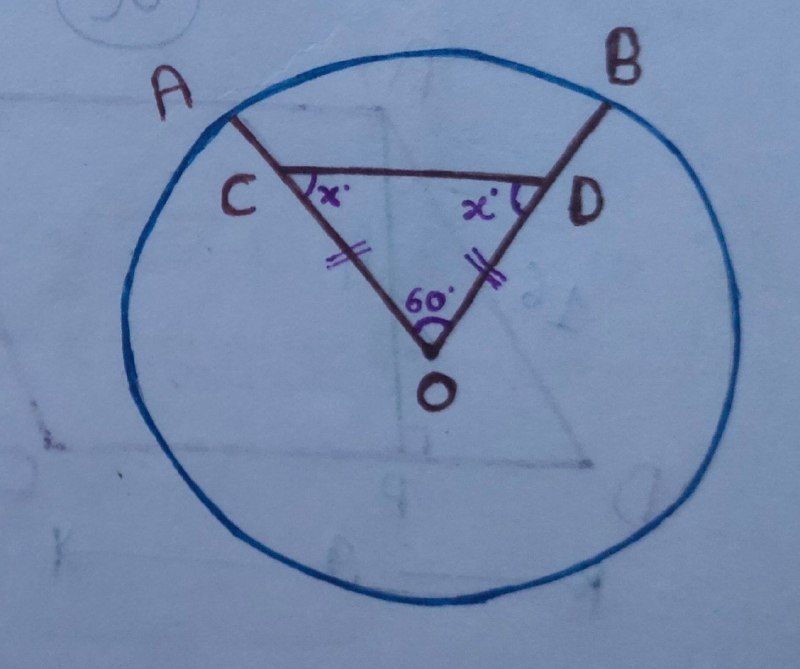
We know that, sum of all the angles of a triangle $ = 180^ {\circ}$
Now, the sum of all the angles of a $ \triangle \text{OCD} = 180^{\circ}$
$ \Rightarrow 60^{\circ} + x + x = 180^{\circ} $
$ \Rightarrow 2x = 120^{\circ} $
$ \Rightarrow x = 60^{\circ} $
So, $ \triangle \text{OCD}$ is a equilateral triangle.
Area of $\triangle \text{OCD} = \frac{1}{2} \text{(area of R)} \quad \longrightarrow (1) $
Area of sector $ = \frac{Q}{360^{\circ}} \times \pi \times (\text{radius})^{2};$ where $Q$ is the angle subtended at the center.
Area of $ R = \frac{60^{\circ}}{360^{\circ}} \times \pi \times (1)^{2} = \frac{\pi}{6} \; \text{cm}^{2} $
Now, the area of $\triangle \text{OCD} = \frac{\sqrt{3}}{4} \; \text{(side)}^{2} = \frac{\sqrt{3}}{4} \; \text{OC}^{2} \; \text{cm}^{2} $
From the equation $(1),$ we get
$ \frac{\sqrt{3}}{4} \; \text{OC}^{2} = \frac{1}{2} \times \frac{\pi}{6} $
$ \Rightarrow \text{(OC)}^{2} = \frac{\pi}{3 \sqrt{3}} $
$ \Rightarrow \text{OC} = \sqrt{\frac{\pi}{3 \sqrt{3}}} = \left( \frac{\pi}{3 \sqrt{3}} \right)^{\frac{1}{2}} \; \text{cm}.$
Correct Answer $: \text{A}$